# Extracting energy and heat from the vacuum, Physical Review E, Vol. 48 No 2, August 1993 from pdf file ‘PREv48_1562.pdf’
Daniel C. Cole (IBM Corporation, Essex Junction, Vermont 05452-4299, Harold E. Puthoff (Institute for Advanced Studies at Austin, Texas 78759-5329) Received 22 March 1993.
Relatively recent proposals have been made in the literature for extracting energy and heat from electromagnetic zero-point radiation via the use of the casimir force. The basic thermodynamics involved in these proposals is analyzed and clarified here, with the conclusion that, yes, in principle, these proposals are correct. Technological considerations for actual application and use are not examined here, however.
PACS number(s): 05.90.+m,03.65.-w,05.40.+j,05.70.-a // 1063-651X/93/48(2)/1562(4)/$06.00, 1993 The American Physical Society
Nearly a decade ago Forward [1] raised the possibility of extracting energy from the alectromagnetic zero-point (ZP) fields that are predicted by quantum electrodynamics to be present in all of space. He described a means of accomplishing this task via a mechanical device consisting of a charged foliated conductor. By using the attractive Casimir force between metal layers to overcome a repulsive electrostatic force, the foliated conductor can be greatly compressed, thereby storing charge at a high electrostatic potential energy.
More recently, one of us (Puthoff) has again raised the possibility of energy extraction from the vacuum [2], while also emphasizing that the energy density of the electromagnetic ZP energy has conservatively been estimated to be equal to or greater than nuclear energy densities [3]. Puthoff suggested a potentially more practical and plentiful means for energy extraction, namely, a method involving a charged plasma. His discussion included the idea of generating heat from the vacuum.
Here we do not comment further on devising practical methods for enabling the vacuum to become a viable, economical alternative to more conventional sources of energy, except to say that, without a doubt, considerable technological effort might need to be expended to adequatly harness such energy. Instead, here we will concentrate on the issue of whether fundamental thermodynamic laws are being violated in even considering this possible source of energy. In particular, certainly the ‘vacuum’ should be considered to be a state of thermal equilibrium at the temperature of T=0. How then can energy be extracted, and even heat generated, at T=0?
Some relatively recent articles by one of us (Cole) provide a starting point for this discussion. These articles analyze the thermodynamics of quasistatic displacement operations on fluctuating electric dipole harmonic oscillators [4-7] and on conducting parallel plates [8]. The operations involve, respectively, the microscopic van der Waals force between atomic systems, and the macroscopic Casimir force between parallel plates. Due to the fundamental thermodynamic definition of T=0, no heat flow should occur at T=0 during quasistatic displacements of these systems. Indeed, for these two systems, as treated via classical physics, only one electromagnetic thermal radiation spectrum was found to ensure that no heat would flow: namely, the classical electromagnetic ZP radiation spectrum, which has the same spectral form as the ZP spectrum predicted to exist via QED. The existence of this radiation results in van der Waals and Casimir forces at T=0, thereby yielding a tight connection between the required spectrum and the resulting forces.
At first thought, a contradiction appears inevitable between the analysis yielding ‘no heat flow at T=0’ and ‘heat extraction at T=0’. However, the contradiction becomes resolved upon recognizing that two different types of thermodynamic operations are being discussed.
The quasistatic operations are thermodunamically reversible, so here no heat flow occurs at T=0. In contrast, the heat generation process discussed in Ref.[2] is thermodynamically irreversible, so heat can be produced, even when the initial temperature is T=0.
The following analysis will cover both the T=0 and T not equal 0 cases. Indeed, although the proposals in Refs.[1,2] discussed only the very idealized T=0 case, they can be shown to be valid also at T not equal 0. Our analysis will use classical physics arguments, as in Refs.[4-8].
The mechanism for heat generation is illustrated in the following thought experiment, which clearly is an impractical process, yet it embodies the necessary points.
Suppose there exists a large number of uncharged parallel plate capacitors. These plates of each capacitor will be attracted to each other by the fluctuating, yet correlated, induced charge distributions in each plate, that arise on account of the fluctuating ZP plus thermal radiation fields. If each pair of plates is allowed to collide, some of the kinetic energy generated will be converted into heat.
Collecting the useable portion of the heat, discarding each pair of plates, and then colliding the next set, in turn, thus yields a means for heat generation. The ‘fuel’ here is the supply of capacitors; the used of capacitors are analogous to the exhaust from gasoline engines or the ‘waste’ from nuclear fuel.
To analyze this process more deeply, the physical description of colliding systems needs to be addressed. In particular, whether we consider macroscopic materials attracted via Casimir forces, or individual atoms attracted together via van der Waals forces, other interatomic interactions need to be considered when the systems come very close to each other. To avoid such complications, let us consider only the situation where, for example, two plates, or two atoms, are initially held apart, then released, and then ‘grabbed’ or blocked by an external force or medium, before the systems collide.
Upon releasing the two attractive systems, they will move toward each other, thereby acquiring kinetic energy. If we then seize them with probes in such a way that the probes should move, or if a material ‘stop’ placed in the path of the system is displaced somewhat, then work will be done upon the probe or stop. Likewise, when making the quasistatic displacements in Refs.[4-8], work could be done on the probes by the displaced systems by making the displacements along the directions of the van der Waals or Casimir forces. In this way, energy is ‘extracted’ by having such systems perform work.
However, heat extraction is quite different than energy extraction, where by energy extraction we henceforth specifically mean the act of having systems perform positive work. During reversible operations, such as are discussed in Refs.[4-8], work will, in general, either be done by or on the systems. However, only for T not equal 0 can heat also be generated during these displacements, where heat, here, consists of energy in the form of electromagnetic radiation that flows from the region surrounding the system in question.
In contrast, for irreversible operations, heat will in general be produced. Upon releasing two plates or particles and then stopping them before they collide, not all of the kinetic energy will typically be transformed into work done upon the material stops. Indeed, in the case of a hypothetical infinitely massive stop, no work will be done. Instead, kinetic energy must be converted into electromagnetic radiation energy. The emitted radiation, or perhaps more appropriately, the radiation that results after interacting with randomizing entities, such as an idealized carbon particle [9,8], would then constitute the heat that flows form the system. In an open system this energy will radiate away and the final state of the system would be the same as if we quasistatically brought the particles together, where work is now done in displacing the material stops that hold the particles.
To show that there are no contradictions with these ideas, we next compare two related thought experiments consisting of an irreversible and a reversible operation, as indicated in Figs. 1(a) and 1(b), respectively. The small darkened circles labeled X and Y represent systems that might be neutral conducting plates, dipole oscillator particles, or other more complicated systems attracted together due to the van der Waals forces. The rectangles with crosshatched lines represent material stops used to hold the systems in place. In Fig. 1(a), three stops labeled A, B, and C are displayed, while in Fig. 1(b), only two stops A and C are shown. The left and right sides of both figures represent the initial time ti and final time tf, respectively, of the two operatins to be discussed.
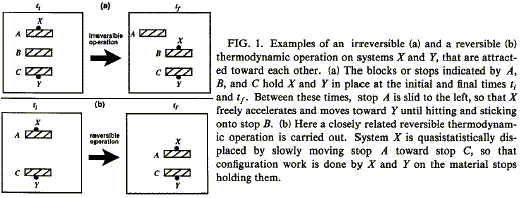
Starting with Fig. 1(a), let stop A be slightly displaced to the left, so that the system is no longer in mechanical equilibrium. A negligible amount of work can be assumed to be done when making this displacement. Subsequently, X will accelerate toward Y and increase its kinetic energy until it hits B. If stop B is not displaced much, or more precisely is 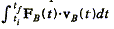 is small, whereFB(t) is the force from X acting on stop B at time t, and vB(t) is the velocity of stop B at time t, then most of the kinetic energy of the system will be converted into electromagnetic energy. If we assume that the box drawn around the system represents a perfectly conducting container that acts to retain all radiation, then this electromagnetic energy cannot escape. Imagining that a small, idealized carbon particule is introduced into the system, then the character of the radiation will again return to a thermal radiation form. The temperature of the system must therefore increase as a result of this sequence.
is small, whereFB(t) is the force from X acting on stop B at time t, and vB(t) is the velocity of stop B at time t, then most of the kinetic energy of the system will be converted into electromagnetic energy. If we assume that the box drawn around the system represents a perfectly conducting container that acts to retain all radiation, then this electromagnetic energy cannot escape. Imagining that a small, idealized carbon particule is introduced into the system, then the character of the radiation will again return to a thermal radiation form. The temperature of the system must therefore increase as a result of this sequence.
if we now carry out a reversible operation in Fig. 1(b) by quasistatically displacing stop A, then systems X and Y arrive in the same position as in Fig. 1(a). Here, however, compression or configuration work was done against the structure holding X in place; i.e., the structural form of the ‘retaining walls’ has changed. In Fig. 1(a), no configuration work was done; the retaining structure remained essentially the same between times ti and tf, except for the infinitesimal displacement of stop A to the left.
To now make the state of the system in Fig. 1(b) the same as the state of the system in Fig. 1(a), the temperature in Fig. 1(b) must be increased. This operation can be done reversibly via sequentially contacting the system with a series of heat reservoirs with infinitesimally increasing temperatures. In this way also, the example in Fig. 1(b) can be used to calculate the net change in entropy for the irreversible process in Fig. 1(a). This change can be found by adding up dQ/T over all the infinite contact operations, where here dQ is a positive heat flow into the box in Fig. 1(b). Since the entropy of the region outside the containing box in Fig. 1(a) does not change, the net entropy change in the universe for the irreversible operation in Fig. 1(a) is therefore positive, in agreement with the second law of thermodynamics.
Figure 2 provides additional insight. The crosshatched lines from states a to c represent the irreversible, adiabatic, free contraction indicated in Fig. 1(a), while the dark line from a to b represents the reversible, adiabatic contraction in Fig. 1(b). To make the two operations end at the same state, namely c, the system in Fig. 1(b) must be reversibly heated, as indicated by the b–>c operation in Fig. 2. We note that if the contraction operation of a–>b is carried out at T=0, then in Fig. 2(c) the a–>b operation would lie at the single point where the Ri and Rf curves come together at T=0, due to the third law of thermodynamics [4,5,8]. Likewise the path a–>b in Fig. 2(b) should become a vertical line at T=0, as will be seen shortly in a specific example.
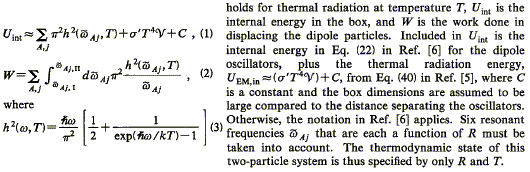
Since the specific shapes of the curves in Fig. 2 depend on the system being analyzed, here we briefly sketch how these curves could be found for a system that can be analyzed in some detail. Consider two dipole harmonic oscillators in a conducting box of volume V, where the oscillators are separated by a distance R that is sufficiently small that the unretarded van de Waals interaction is dominant. Hence, the expressions in Ref. [6] apply:
To simplify the expressions further, if we assume the oscillators are separated along the x direction and are constrained so that oscillations occur only along the y direction, then only 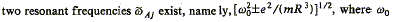 is the natural resonant frequency of the oscillating particle’s motion, and e and m are the charge and mass, respectively, of this same particle.
is the natural resonant frequency of the oscillating particle’s motion, and e and m are the charge and mass, respectively, of this same particle.
Knowing Ra, Ta, and Rc, then Tc can be found by considering the irreversible adiabatic free contraction and applying, conservation of energy: Uint(Ra, Ta) = Uint(R,sub>c, Tc). To find Tb, with Rb=Rc, we can start from state a and follow the adiabatic reversible path a–>b by demanding that dUint(R,T)=(dW/dR)dR along each infinitesimal part of the path. One obtains
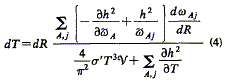
which can then be integrated to find Tb. As anticipated from Refs. [4] and [6], if T=0 at a, then dT=0 from Eqs. (3) and (4) for any piece of this path from a–>b, thereby resulting in the a–>b path in Fig. 2(b) becoming a vertical line at T=0. Finally, Td can be found by starting from Tc and integrating along the reversible, adiabatic path from c–>d.

References:
[1] R.L. Forward, Phys. Rev. B30, 1700(1984)
[2] H.E. Puthoff, Specul. Sci. Technol. 13, 247 (1990)
[3] R.P. Feynman and A.R. Hibbs, Quantum Mechanics and Path Integrals (Mc Graw-Hill, New York, 1965), p.245;
see also C.W. Misner, K.S. Thorne, and J.A. Wheeler, Gravitation (Freeman, San Francisco, 1973), p. 1202ff.
[4] D.C. Cole, Phys. Rev. A 42, 1847 (1990).
[5] D.C. Cole, Phys. Rev. A 42, 7006 (1990).
[6] D.C. Cole, Phys. Rev. A 45, 8953 (1992).
[7] D.C. Cole, in Formal Aspects of Electromagnetic Theory, edited by A. Lakhtakia (World Scientific, Singapore, 1993)
[8] D.C. Cole, Phys. Rev. A 45, 8471 (1992).
[9] M. Planck, The Theory of Heat Radiation (Dover, New York, 1959). This publication is an english translation of the second edition of Planck’s work entilted Waermestrahlung, published in 1913. A more recent republication of this work is The Theory of Heat Radiation, Vol. 11 of The History of Modern Physics and Astronomy (AIP, New York, 1989).
# ALTERNATIVE ENERGY SOURCES: Good News/Bad News and “The 1-Watt Challenge”, H. E. Puthoff, Ph.D., from pdf ‘1-WattChallenge[1].pdf’
Institute for Advanced Studies at Austin, Austin, Texas. Presented at: International Symposium on New Energy, Denver, Colorado, May 12-15,1994
ABSTRACT
In researching innovative energy sources, we are faced with a good news/bad news situation. On the good news side, new arenas of research activity are being opened up and pursued vigorously. These range from relatively mainstream approaches to develop solar energy, to highly innovative approaches to extract energy from vacuum fluctuations. On the bad news side, despite varying degrees of claimed success, there are as yet no standalone devices in this class (with the exception of solar devices) that unambiguously demonstrate the generation of net excess energy to the satisfaction of the consens ual research community. It is suggested here that the credibility of these efforts requires meeting what we call “The IWatt Challenge,” the demonstration of a device that can continuously generate, on a stand-alone, self-powered basis, a minimum of at least I watt excess average output power.
Background
In the field of alternative energy research, researchers are attempting to develop energy sources based on the application of innovative concepts that, for the most part, lie outside the mainstream of energy research and development. These range from the relatively straightforward techniques of capillary fusion, through the controversial phenomena of “cold fusion,” to the speculative proposals to extract energy from vacuum fluctuations via Casimir processes, or the claims of energy generation from the very fabric of space itself via rotating magnetic devices (e.g., by Faraday-disk homopolar generator action). Given the overused but nonetheless useful phrase “extraordinary claims require extraordinary proof,” we comment here on the difficulties encountered in these efforts, both in gaining scientific credibility and in obtaining appropriate high- risk investment capital, and suggest a strategy to meet these challenges.
The Good News
The good news is that there is theory and evidence, even demonstration, acceptable to the mainstream scientific community, that new, alternative potential energy resources exist that have yet to be brought to fruition. In addition to the mainstream examples of solar energy and thermonuclear fusion, capillary fusion and Casimir energy extraction come to mind. Thus the innovative energy field as a field is not pseudoscience, or the pursuit of a chimera. What remains to be proven, however, is whether the fundamental processes involved can be brought from proof-of -principle to engineering maturity so as to constitute market-viable energy resources.
As an example, consider the case of so-called vacuum zero-point energy (ZPE), a research area being pursued, both theoretically and experimentally, by our research team at the Institute for Advanced Studies at Austin. This research is based on the fact that, in accordance with the discoveries of quantum theory, empty space is not truly empty, but rather contains an enormous amount of untapped electromagnetic energy known as the zero-point energy, or ZPE [1]. (The adjective “zero-point” signifies that such energy exists even at a temperature of absolute zero where no thermal effects remain.) Such energy can be traced to radiation from the fluctuating quantum motion of charged particles distributed throughout the universe [2]. Well-known physical consequences of the ubiquitous background ZPE include the perturbation of atomic spectral lines known as the Lamb Shift, the van der Waals forces of chemical attraction at absolute zero, and the Casimir Effect, a unique attractive quantum force between closely-spaced metal or dielectric plates, or other geometries.
Since the energy associated with the ZPE is known to be essentially ubiquitous and inexhaustible, the question that arises is whether such energy can be “mined” for practical use, that is, extracted to perform useful work. Although it would be natural to assume that any attempt to extract energy from the background ZPE might somehow violate energy conservation laws, or at least thermodynamic constraints, a careful analysis shows that this is not the case, and that energy and heat can in principle be extracted without violation of fundamental precepts [3]. As discussed in the literature, just such processes might already occur in Nature in certain large-scale, energetic astrophysical phenomena [4].
With regard to laboratory experimentation, the candidate mechanism for energy extraction is the above-mentioned Casimir Effect, the ZPE-driven attractive force between closely-spaced plates. This attractive force can be shown to be due to partial shielding of the region between the plates from the background ZPE, with the consequence that the plates are driven together due to the resulting imbalance in ZPE radiation pressures [5]. As emphasized by Forward at Hughes Research Laboratories in his paper “Extracting Electrical Energy from the Vacuum…,” proof-of -principle of Casimir energy extraction is seen during the process of the plates moving together, which results first in the conversion of the attractive Casimir (vacuum) potential energy into kinetic energy, then heat as the plates collide [6]. In an alternative embodiment envisioned by Forward, the plates are electrically charged with the same-sign charge, resulting in the buildup of electrical (Coulomb) energy as the stronger attractive (1/d4) Casimir force overcomes the weaker Coulomb repulsion at small spacings and draws the plates together. While these mechanical examples are admittedly impractical for significant, continuous energy generation, they nonetheless demonstrate the basic principle involved.
Experimentation in our laboratory is directed toward a plasma version of the above process. In short, we are investigating the possibility of a Casimir-type pinch effect that may be a contributing mechanism to the generation of high-density charge clusters in micro-arc discharges (which itself has led to the development in our laboratory of a new, patented microelectronics technology known as condensed-charge technology, CCT).
With regard to the potential energy extraction process of interest here, we envision a “Casimir fusion” process, which in its cycle of operation would mimic the nuclear fusion process, but without the radioactive byproducts. it would begin, like its nuclear counterpart, with an initial energy input to a plasma to overcome a Coulomb barrier, followed by a condensation of charged particles drawn together by a strong, short-range attractive potential (in this case a Casimir rather than a nuclear potential), and with an accompanying energy release in some form (heat, electrical). Should the energy requirements for plasma formation, and electrical circuit and other heat losses be kept at a level below that required for break-even operation, then, as in the nuclear case, net useful energy would be generated. Calorimetry measurement of possible excess heat (energy) generation in this process is ongoing in our laboratory. Although encouraging results, both by calorimetry and electrical measurement, have been obtained under certain conditions at various times, stand-alone operation, the sine qua non of proof-of- utility (as will be argued below) has not yet been achieved.
In addition to the scientific soundness of new energy generation principles, as a separate “good-news” item we have had the opportunity to sample the pulse of the oil industry, and of the government, as to their potential response to the development of alternative energy sources as discussed here. Contrary to the prevailing “folk-myth” of some, we have found little evidence of potential suppression.
With regard to the oil industry, for example, we briefed the presidents, vice-presidents or research directors of Pennzoil, Texaco, Tenneco, Marathon Oil and Coastal Oil. Without exception, it appeared that the development of alternative energy sources would be welcomed for the simple reason that if the burden of major energy use were to be removed from the oil industry, then their rapidly dwindling resource could be conserved for a longer period of time, and they could concentrate on the development of pharmaceuticals, plastics, synthetic fibers, etc., for which the profit margins are significantly greater. One executive likened the present use of oil for gross transportation and utility requirements to “heating one’s house by burning Picassos and Van Goghs,” and opined that the oil industry would itself become a major user of new energy technologies to increase efficiency and reduce cost in refinery operations.
Similarly, in briefing various government agencies, including the DOD, NIST (National Institute of Standards and Technology, formerly the National Bureau of Standards), and the Patent Office, we did not encounter any evidence of suppression or hindrance of our efforts, only encouragement.
The Bad News
Despite the fact that a number of experimenters, including ourselves, feel that steady (sometimes not so steady!) progress is being made toward the goal of new, viable alternative energy sources, we must face the fact that an unambiguous demonstration of a working model remains elusive. Facetiously, I would say that by unambiguous I mean sufficiently riveting that one must turn away any further potential investors. Seriously, by unambiguous I think we require a broad consensus that the device under consideration (a) exhibits a non-borderline excess useful energy output not traceable to ordinary, mundane sources, based on close scrutiny by independent observers (nondisclosure agreements acceptable); (b) said excess energy is measurable by standard measurement apparatus operating within standard operating characteristics; (c) some plausible concept of the energy gain mechanism is proffered; (d) a reasonable requirement for independent reproducibility and replicability is met; and (e) I suspect that stand-alone, self-powered operation (as opposed to energy-out/energy- in gain measurement) is required a potentially contentious point I will defend below. Many would say that the Swiss ML converter satisfies (a) and (b) somewhat, (e), but not (c) and (d); “cold fusion” satisfies (a), (b) – (d) somewhat, but not (e); and so forth.
Although each of the above points could stand detailed discussion, I will “cut to the chase” and argue that, in my opinion, in alternative energy research (e) is the most critical. If proof of a viable process requires separate measurement of input and output energies, and a comparison of same, then arguments can always be raised concerning the measurement procedures, and not just by intractable skeptics. For one, as research in the “cold fusion” arena has shown, calorimetry is as much of an art as it is a science. In our own laboratory calorimetry efforts, for example, which have involved sophisticated, computer-automated apparatus, we are many years and tens of thousands of dollars into nth- generation modifications. This even includes detailed evaluation and eventual rejection of commercial calorimeters as inadequate to the task. Again, as George Hathaway discusses elsewhere in this conference, electrical me asurement can be problematical for circuits involving, for example, high- intensity spark-discharge phenomena where stray capacitance and inductance effects can predominate over expected resistance characteristics of a load for pulse signals in the nanoseconds range if, on the other hand, one has a modicum of excess energy, one can in principle tailor whatever portion of the output energy is required to provide input driving, head-to-tail, so to speak, to achieve stand-alone operation; then arguments concerning measurement become moot. There are, of course, justifiable reasons along the way as to why this stringent requirement cannot be met. In our own efforts, for example, outputs in the form of heat, even with gain, cannot survive the inefficiencies of thermoelectric conversion to provide a required high- voltage DC input. Thus we too cannot yet meet this requirement, but it is a stated goal of our research effort. In those cases where multiples of input energy are claimed, however, nothing will show up an error in measurement, if there is one, as fast as an attempt to run head-to-tail; and, conversely, nothing will validate a true energy gain as quickly, either.
Other arguments that could be raised against requirement (e), however, we find not so compelling. For example, it has been offered that self-excitation smacks of “perpetual motion,” and that the consequences of this appellation (e.g., difficulties with the patent procedure) argue against its use. In fact, “perpetual motion of the second kind,” in which conversion of energy from some source offsets losses in a system (which presumably is a condition that must be met in a “free energy” machine unless we were to discover new evidence to the contrary), is perfectly acceptable in physics and engineering. (The “perpetual motion” of Niagara Falls is a good example, where the sun provides the energy we do not have to pay a price for, through the evaporation/rain cycle.) In the case of our own research, for example, we have been successful in obtaining patents that speak of the conversion of ambient vacuum energy, as opposed to the generation of “free” energy.
Another argument we have heard is that one must provide clearly usable amounts of power (e.g., kilowatts) to have a viable energy technology. It is claimed that the diversion of a significant amount of output power to self-excite (as opposed to the use of, say, a separate low-power input) would be self-defeating in this regard, demonstration of practical utility would suffer, investment would not be as forthcoming, etc. We would offer that the elimination of measurement ambiguity would more than offset the downsizing of a prototype demonstration device in the eyes of any potential realistic investor.
Finally, adherence to requirement (e) eliminates potentia l confusion attendant to the discussion of “incremental” as opposed to “net” gain, a difficulty often faced in attempting to evaluate, for example, homopolar- generator devices. Again, we have ourselves dealt with this problem in our own laboratory in which a homopolar generator is seen to “waste” a certain number of watts just to overcome windage and friction, but when loaded does not appear to require as much incremental input as is being generated at the output. It is tempting to extrapolate that engineering improvements to reduce the waste “tare” will result in over-unity efficiency, but one must demonstrate that indeed this is the case, since motor efficiencies typically change radically with changes in operating parameters.
“The 1-Watt Challenge” Strategy
Based on the above discussion, we would recommend that the surest route to credibility for alternative energy research lies in meeting what we call “The 1-watt Challenge.” This is the demonstration of a device that, on a stand-alone, self-powered basis, can continuously generate a minimum of at least I watt excess average output power.
Specifically, consider that one had a device that required ten watts of input power from an external source, say, a battery, but with this input was capable of generating, say, twenty-one watts of output power in the form of heat (a little over 2:1 power gain). We would argue that if one could operate alternatively by diverting twenty of those output watts through a 50%-efficient heat-to-electric converter to provide the ten-watt input power, the reduction of the output from twenty-one watts to one watt would be worth the sacrifice in output power to remove the ambiguity of the measurement argument, and the reliance on a separate energy source. Clearly, since to our knowledge such operation has not yet been demonstrated to consensual satisfaction, this is a tough requirement to meet, despite the perhaps disappointingly-small-sounding, 1-watt requirement. Nonetheless, in the absence of our research community collectively “ho lding its feet to the fire” to meet such a challenge (and this includes our own research effort as well), we would submit that the credibility of the alternative energy research field is subject to erosion by false hopes and unsubstantiated claims. Alternatively, the satisfaction of such a requirement would provide a solid foundation for discussion and presentation of the reality of the energy developments we wish to bring to fruition. And this is a challenge I think can be met.
REFERENCES
– 1. H. E. Putho ff, “The Energetic Vacuum: Implications for Energy Research,” Spec. in Science and Tech. 13, 247 (1990).
– 2. H. E. Puthoff, “Source of Vacuum Electromagnetic Zero-Point Energy,” Phys. Rev. A 40, 4857 (1989); 44, 3385 (1991).
– 3. D. C. Cole and H. E. Puthoff, “Extracting Energy and Heat from the Vacuum,” Phys. Rev. E 48, 1562 (1993).
– 4. A. Rueda, “Survey and Examination of an Electromagnetic Vacuum Accelerating Effect and its Astrophysical Consequences,” Space Science Reviews 53, 223 (1990).
– 5. P. W. Milonni, R. J. Cook and M. E. Goggin, “Radiation Pressure from the Vacuum: Physical Interpretation of the Casimir Force,” Phys. Rev. A 38, 1621 (1988).
– 6. R. L. Forward, “Extracting Electrical Energy from the Vacuum by Cohesion of Charged Foliated Conductors,” Phys. Rev. B 30, 1700 (1984).
# SETI, the Velocity-of-Light Limitation, and the Alcubierre Warp Drive: An Integrating Overview from pdf ‘SETI.pdf’
(Physics Essays, Vol. 9, No. 1, pp. 156-158, 1996) H.E. Puthoff, Ph.D.
Abstract
In SETI (Search for Extraterrestrial Intelligence) conventional wisdom has it that the probability of direct contact by interstellar travel is vanishingly small due to the enormous distances involved, coupled with the velocity-of- light limitation. Alcubierre’s recent “warp drive” analysis [Class. Quantum Grav. 11, L 73 (1994)] wit hin the context of general relativistic dynamics, however, indicates the naivete of this assumption. We show here that Alcubierre’s result is a particular case of a broad, general approach that might loosely be called “metric engineering,” the details of which provide yet further support for the concept that reduced-time interstellar travel, either by advanced extraterrestrial civilizations at present or ourselves in the future, is not, as naive consideration might hold, fundamentally constrained by physical principles.
Key words: SETI, velocity of light, general relativistic dynamics, space-time metric, interstellar travel, vacuum energy, Casimir effect, vacuum engineering, warp drive, superluminal travel
SETI researchers routinely subscribe to the view that interstellar travel between civilizations is exceedingly improbable due to the velocity-of-light limitation, with but few dissenting views offered.(1,2).
Hence there has evolved, on the one hand, the emphasis on searches of the electromagnetic spectrum for information-bearing signals and, on the other, the reasoned dismissal by the scientific community of any evidence purported to be a signature of extraterrestrial visitation. (3)
As shown recently by Alcubierre, however, rejection of the concept of hyperfast (superluminal) travel is not justified when one takes into account the possibility of engineered dynamic space-times within the context of general relativity. (4) Specifically, Alcubierre showed by example that by distorting the local space-time metric in the region of a spaceship in a certain prescribed way, it would be possible to achieve motion faster than the speed of light as seen by observers outside the disturbed region, without violating the local velocity-of- light constraint within the region.
Furthermore, the Alcubierre solution shows that the proper acceleration along the spaceship’s path would be zero and the spaceship would suffer no time dilation, features presumably attractive in interstellar travel. We present here a supporting viewpoint that further explicates the Alcubierre approach as a special case of an overarching concept of metric engineering that can be stated in an especially compact form, fully incorporating general relativistic dynamics.
To elaborate the metric engineering perspective, we begin with the apparent velocity-of- light limitation. As a physical concept this limitation is based on the fact that mass and energy find mathematical expression in a form proportional to 1/[l-(v/c)2]1/2, which implies that an infinite amount of energy would be required just to accelerate a mass to the velocity of light v = c. A hidden assumption in the argument that this constitutes a practical limitation with regard to interstellar travel, however, is the idea that the value c is a fixed, immutable constant of nature, understood in a straightforward, natural way. It is this crucial assumption that is called into question and redefined, however, by the metric engineering approach.
In engineering terms the velocity of light in free space c is given by the expression c = 1/(µ0 0)1/2, where in mks units µ0 = 4 x 10-7 H/m and 0 = 8.854 x 10-12 F/m, are, respectively, the magnetic permeability and dielectric permittivity of the vacuum. Therefore, the argument that c is fixed is, at base, an argument that µ0 and 0 are fixed and not subject to manipulation by technological means. If, on the other hand, these vacuum constants were subject to change such that within a localized region the value c could be made to assume a new value, say c’ = 10c, then, without violating the governing equations of physics, travel at speeds greater than the conventional velocity of light would be possible; it is just that a new restriction would apply involving the (elevated) local velocity of light, and travel inside the local light cone would still obtain, a point demonstrated in detail in the Alcubierre example.
Although perhaps surprising to the nonspecialist, within the context of general relativity and vacuumenergy physics, such variability of the free space velocity of light c (as seen from a distant frame) under certain conditions has long been part of the literature. For the case of propagation near a massive body, for example, we have a reduction in the velocity of light by an amount proportional to the gravitational potential, a result first noted by Einstein himself. (5) For the case of propagation between closely spaced conducting boundaries as in discussions of the Casimir effect, we have an increase in the velocity of light which is associated with the reduction of vacuum fluctuation energy between the plates. (6) In short, as emphasized by Wesson, the speed of light c is context-dependent and not as fundamental as widely believed.(7)
Such variations in c, considered in terms of its subcomponents µ and , are routinely treated in a compact form that recommends itself for simplicity of concept, the so-called “TH µ” formalism used in comparative studies of gravitational theories.1 This approach has its foundation in the recognition that the covariant Maxwell equations in a Riemannian space with arbitrary metric are identical in form with the usual vector Maxwell equations for a material medium with variable and µ, where these parameters are themselves now a function of the metric.(8) This concept can be extended to nonmetric theories as well, and in the TH µ context goes under the name “gravitationally modified Maxwell equations. “1 The formalism is then completed by casting the Lagrangian for particle motion under the influence of electromagnetic and gravitational fields into a canonical form involving two additional metric-dependent functions, T and H. Such a formalism leads naturally to the concept of metric engineering in which one’s familiarity with variable -µ media can act as an intuitive guide. 3,(8) Although under ordinary conditions effects involving variations in vacuum values of µ, , and hence c typically are vanishingly small, they nonetheless indicate the possibility under extraordinary conditions of “vacuum engineering,” as Nobel Laureate T. D. Lee put it.(9) The Alcubierre warp drive example, which can be reframed within the TH µ context, is an especially pithy example of such, and additional space-times with desired properties can be derived at will within this context. 4
Therefore, the proper conclusion to be drawn by consideration of engineered metric/vacuum-energy effects is that, with sufficient technological means to appear ‘magic” at present (to use Arthur C. Clarke’s phrase characterizing a highly advanced, technological civilization), travel at speeds exceeding the conventional velocity of light could occur without the violation of fundamental physical laws. And, we might add, this could in principle be done without recourse to concepts as extreme as wormhole traversal. (10) (However, clearly, exotic matter/field states, e.g., macroscopic Casimir- like negative-energy-density vacuum states, would be required.) As a result, the possibility of reduced-time interstellar travel, either by advanced extraterrestrial civilizations at present or ourselves in the future, is not fundamentally constrained by physical principles.
Acknowledgment
I wish to acknowledge the Fetzer Institute in Kalamazoo, Michigan, for their support of this work.
Endnotes
– 1 A.P. Lightman and D. L. Lee, Phys. Rev. D 8, 364 (1973). See also, C. M. Will, Phys. Rep. 113, 345 (1984) for a later overview perspective. Extens ions of the Lightman and Lee approach (point charges interacting classically with electromagnetic and gravitational fields) to include quantum mechanical analysis of atomic clocks and the standard model of fundamental (electroweak and strong) interactions are given in, respectively, C.M. Will, Phys. Rev. D 10, 2330 (1974) and J.E. Horvath, E.A. Logiudice, C. Riveros, and H. Vucetich, Phys. Rev. D 38, 1754 (1988).
– 2 In the TH µ approach the functions T and H are introduced by requiring that the Lagrangian for the motion of charged particles under the joint action of gravity and the electromagnetic field Ai be expressed in the canonical form
L = Ldt = [ -m0(T – Hv 2)1/2 + eAi v i]dt,
where T and H, as well as and µ, are functions of the metric, that is, of a gravitational potential U. For standard theory of interest in this note (a metric theory), the four functions TH µ are related by = µ = (H/T)1/2. Although for ease of application in comparing a broad range of gravitational theories (e.g., scalar, vector, tensor, scalar-tensor, metric, and nonmetric) the required Lagrangian form is typically met by restricting consideration to static, spherically symmetric gravitational fields, Lightman and Lee emphasize that the TH µ approach is sufficiently general that all the results obtained can be shown to hold “even if U is an arbitrary but time- independent function of position.”1 Thus for a well-behaved standard metric type of theory of interest here, generation to nonsymmetric conditions can be carried out on a case-by-case basis without undue difficulty.
– 3 For a detailed and explicit discussion of the isomorphisms between variable -µ media and general relativistic (metric) theories, see R.H. Dicke, Rev. Mod. Phys. 29, 363 (1957). See also as modified and corrected in R. H. Dicke, “Mach’s Principle and Equivalence,” Proceedings of the Intentional School of Physics “Enrico Fermi” Course XX, Evidence for Gravitational Theories, edited by C. Moller (Academic Press, NY, 196 1), p. 1.
– 4 A detailed examination of the Alcubierre warp drive example within the TH µ-type framework is in preparation (to be published).
References
– 1. T.B.H. Kuiper and M. Morris, Science 196, 616 (1977).
– 2. J.W. Deardorff, Q. J. R. Astron. Soc. 27, 94 (1986).
– 3. See, for example, F. Drake and D. Sobel, Is Anyone Out There? The Scientific Search for Extraterrestrial Intelligence (Delacorte, NY, 1992).
– 4. M. Alcubierre, Class. Quantum Grav. 11, L73 (1994); see also I.A. Crawford, Q. J. R. Astron. Soc. 36, 205 (1995).
– 5. A. Einstein, Ann. Phys. 35, 898 (191 1).
– 6. K. Scharnhorst, Phys. Lett. B 236, 354 (1990).
– 7. P. Wesson, Space Sci. Rev. 59, 365 (1992).
– 8. A.M. Volkov, A.A. Izmest’ev, and G.V. Skrotskii, Sov. Phys. JETP 32, 686 (1971).
– 9. T. D. Lee, Particle Physics and Introduction to Field Theory (Harwood Academic, London, 1988), p. 826.
– 10. M. Morris, K. Thorne, and U. Yurtsever, Phys. Rev. Lett. 61, 1446 (1988).
H.E. Puthoff, Institute for Advanced Studies at Austin, 4030 Braker Lane W., #300, Austin, Texas 78759-5329 U.S.A., puthoff@aol.com